2. Interaction between two CA

The picture depicts four CA histories driven by rule #357. The first (marked by a 0), depicts a history of a single CA , which gradually grows (downward), yet will soon die. The next history depicts two CAs whose seeds were planted 5 units apart from each other. After fusing, they became immortal. The next history depicts two CAs whose seeds were 22 units apart from each other. Both grow along each other, and shortly before they die they interact (fertilize) each other and become immortal. In the last history the two CAs interact, gain mass (strength), and die.


click here to read more

1. First steps

In his book, ''A New Kind of Science" (1), Stephen Wolfram describes a new modeling tool, called Cellular Automat (CA). Simple programs evolve in an unpredicted fashion and become extremely complex. CA is particularly suitable for illustrating some characteristics of life, which cannot be modeled with other Artificial Life (AL) tools, e.g., neural networks (NN), or genetic algorithms (GA).

Life is complex, creative , optimal , and continually moves (changes). These characteristics will be illustrated here with CAs . specified by Wolfram. The following examples will apply two totalistic CAs with the respective rules , #357, #600. The first image illustrates the structure of a rule #600 CA. It originates in a seed which is always a 1. Each row represents a state of the CA. The last row is its present state. The picture depicts a CA trajectory which is also its history.

Click here to continue

Two kinds of models

There are two kinds of models, centralized or top-down, and distributed, or bottom-up models. Most physical models are of the first kind. They are governed by top-down laws that control entire systems. None of these suffices to describe even the simplest organism, which is complex and its properties emerge. Traditional mathematical tools fail to untangle life's complexity. We may distinguish between two kinds of complexity linear and non linear. Only the first can be resolved with traditional mathematical tools like logic, or induction. Life's complexity is non linear.

Life is an oriented change.

Like a river that flows in one direction. Yet even a river could not serve as an adequate model for life, since its water is carried to the sea as such and does not change, while the ingredients of life continually transform. Fire might be regarded as best metaphor for life. It is born in the burning wood. As it raises upward, its color continually changes, from yellow to red, and blue. None of Artificial Life (AL) models can simulate a fire, neither a river, and yet some serious scientists claim that these simplistic models are a form of life, life in silico.

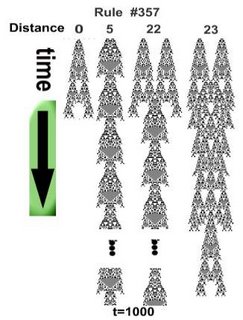
Cellular automata

S. Wolfram's book "A New Kind of Science" is an excellent introduction to cellular automata (CA). Yet it lacks two basic ingredients of life. His CA are infinite and immortal, while life is not. They consist of simple geometrical structures like triangles, while life is amorphous. Above all CA lack an essential ingredient of life, oriented turnover.(streaming). Why not augment CA so as to portray this property of life?

Click here to learn more